Pump related formulas | Power calculation, Total Head, NPSH, Affinity laws

In this article provided pump related formulas like fluid flow rate and velocity, power calculation, Specific Speed of Pump (Nq), Total Head, Pump Torque and temperature rise, Net Positive Suction Head, Affinity laws for pump, Pump Efficiency & Overall Efficiency of the Pump

Basic required formulas for the operation of Pump

Formulas regarding product while pumping

Volume of the fluid (Q )

$Volume \ of \ fluid \ (l/sec)= \frac{ Mass \ flow \ rate \ (kg/sec) \ \time 1000 }{ Density \ (kg/m^3) }$

Velocity of the Fluid ( V )

$V= \frac{ Q }{ A }$

Here

V = Velocity of fluid in m/sec
Q =Volume of Fluid (m³/sec)
A = Pipe line area (m²)

$Velocity \ of \ fluid \ in \ pipe = \frac{ Discharge \ Flow \ Rate \ ( m^3/hr)}{ \frac{\pi}{4} \ \time \ Pipe \ ID (m) \time \ 3600}$

$V= \frac{ Q \time 353.6}{ D^2 }$

V = Velocity of fluid in m/sec
Q =Volume of Fluid in m³/hr
A = Pipe line dia in mm

Reynolds Number of the fluid

$R_e= \frac{ D \ \time V \time \rho }{ \mu }$

Here
D = Dia of the tube in meters
V = fluid velocity in m/sec
ρ= density of the fluid (kg/m³)
μ = Absolute viscosity in Pas

Absolute viscosity of the fluid (μ )

μ = υ x ρ

where:
υ = Kinematic viscosity (mm²/s)
ρ = density of the fluid in kg/m³

μ = Absolute viscosity of the fluid in mPas

Darcy Friction Factor

$f_d = \frac{ 64 }{ R_e}$

where:
f_d = friction factor (Darcy)
Re = Reynolds number

Pump Power Calculation

Hydraulic Pump Power

The ideal hydraulic power to drive a pump depends on liquid density , differential height to lift the material and flow rate of the material.

$Hydraulic \ Power H_p = \frac{Q \ H \ \rho \ g }{1000}$

Here

Hydraulic power in watt

Q = Flow rate in M³/sec

H = Total head in meters = Discharge head + Suction head

ρ = Density of the Fluid kg/dm³ (1 kg/m³ = 0.001 kg/dm³)

g = Acceleration due to gravity (m/sec²)

Pump Power input or Pump shaft Power

The pump power input of a centrifugal pump is the mechanical energy at the pump coupling or pump shaft absorbed from the drive.

$Pump \ Shaft \ Power = \frac{ Hydraulic \ Power }{ Pump \ \ Efficiency}$

$Pump \ Shaft \ power \ = \frac{Q \ H \ \rho \ g }{1000 \time \ \eta_p }$

Here

Q = Flow rate in M³/sec

H = Total head in meters = Discharge head + Suction head

ρ = Density of the Fluid ( kg/dm³)

η_p =Pump efficiency

$Pump \ Shaft \ power \ = \frac{Q \ H \ \rho \ g }{367 \time \ \eta_p }$

Here

Q = Flow rate in M³/hr

H = Total head in meters = Discharge head + Suction head

ρ = Density of the Fluid ( kg/dm³)

η_p =Pump efficiency

Pump input power from pump shaft power

$Electrical \ Input \ Power = \frac{ Pump \ Shaft \ Power }{ Motor \ \ Efficiency \ ( \eta_m) }$

Pump input power from current and voltage

$Pump \ input \ power \ from \ current & voltage = \frac{ Current (A) \time Voltage (V) \time \ Motor Efficiency (\eta_m) \time \ Pump Efficiency (\eta_p) }{ 57735}$

Here all efficiencies are in decimals

Specific Speed of Pump (Nq)

Specific Speed of pump (Nq) is identifies the geometrical similarity of pumps. It is useful to comparing different pump designs irrespective of pump size

Nq = $\frac{3.65 \ \times \ N \ \times \ Q \ ^{0.5}}{H \ ^{0.75}}$ = $\frac{ 333 \ \times \ n \times \ \sqrt{Q}}{(g \times H ) \ ^{3/4}}$

Where Nq = Dimensionless parameter

N = RPM of pump

n = Rev/sec of Pump

Q = Flow rate in m³/sec

H = Head in meters

g = Gravitational constant ( 9.81 m/sec²)

Suction Specific Speed at best efficiency point

$N_s = \frac{ N \times \ \sqrt{Q}}{(NPSH ) \ ^{3/4}}$

Ns = Suction Specific speed of pump (Dimensionless parameter)

N = RPM of the pump

Q = Flow rate in M³/hr

NPSH = Net positive section head in meters

Total Head

In pumping system, Head means it is a height of a liquid coloumn.

In vertical pipe any liquid coloumn of water exerts a certain pressure (force per unit area) on a horizontal surface at the bottom area, this pressure is expressed in metres of liquid column or kg/cm².

H = H_t – (±H_s)

where:
H_t = total discharge head
H_s = total suction head

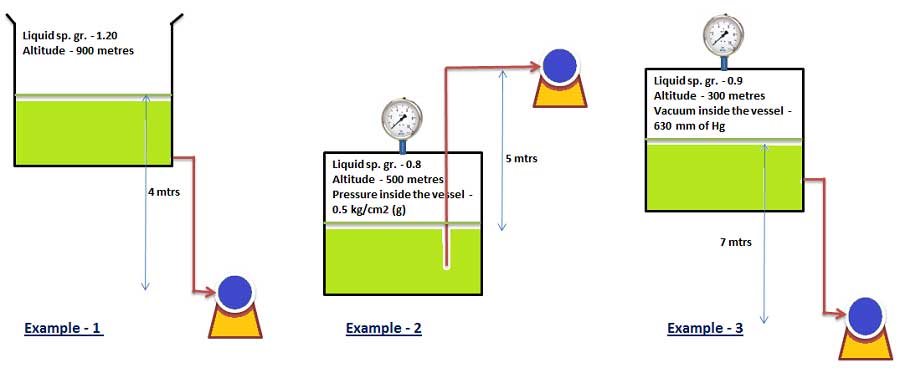
Total Discharge Head

H_t = h_t + h_ft + p_t

where:
h_t = static discharge head
h_ft = pressure drop in discharge line

p_t = Pressure head in delivery
p_t > 0 for pressure
p_t < 0 for vacuum
p_t = 0 for open tank

Total Suction Head

H_s = h_s + h_fs + (± p_s)

where:
h_s = static suction head
h_f_s = pressure drop in suction line

p_s = Pressure head in suction
p_s > 0 for pressure
p_s < 0 for vacuum
p_s = 0 for open tank

Pressure Head for Pump

Pressure Head of the pump suction must be considered according to the condition of source tank.

Pressure head calculated as per pumping system source tank is under some gauge pressure or vacuum open or open to atmospheric than pressure head is calculated in metres of water column (MWC) of Feet of water column of liquid.

$Head ( meters) = \frac{ 10.2 \time P (bar) }{ Specific \ Gravity }$

Velocity head

$H_v \ ( \ in \ m) = \frac{ V^2 }{ 2g} \ = 0.0510 (V)^2 = \frac{6380(Q)^2}{d^4}$

Here Hv = Velocity head in meters

V = Fluid velocity in m/sec

g = Gravitational constant (9.81 m/s²)

Q = Flow rate in m³/hr

d = pipe inside diameter in mm

Shutoff head :

Shutoff head of the centrifugal pump is the maximum head that can be developed by a pump operating at a set speed

Please go through the below link for more information about pump head

Pressure Head | Velocity head | Static Suction Head Calculation of PUMP

Pump Torque and temperature rise

$Pump \ Torque \ (in \ N-m) = \frac{ 9450 \time Pump \ Power (kW) }{ Pump \ Speed (rpm)}$

Temperature rise in pumps can be calculated as per the below formula

$\Delta T = \frac{ P \ (1- \eta_p)}{C_p \ \time \ Q \time \ \rho}$

Here

ΔT = Temperature rise in the pump (in ^oC)

P = brake power (kW)

ηp =Pump efficiency

Cp = specific heat of the fluid (kJ/kg ^oC)

Q = Flow rate of the pump (m³/second)

ρ = fluid density (kg/m³)

Net Positive Suction Head

Net Positive Suction Head Required (NPSHr ):

The amount of NPSH the pump requires to avoid cavitation is called Net Positive Suction Head Required (NPSHr). This value of the pump is determined based on actual pump test by the vendor.

Net Positive Suction Head Available( NPSHa) :

Net positive suction head available is the difference between the saturation pressure and the pump suction pressure for the liquid being pumped.

The amount of Net positive suction head available (NPSHa) to the pump from the suction line is termed NPSHa.

NPSHa = Absolute Pressure head + Static head (difference in elevation) – Vapor pressure head – Friction head loss in the piping, valves and fittings.

Net positive suction head available must be greater than or equal to the net positive suction head required to avoid cavitation. It can be stated mathematically as shown below.
NPSH a ≥ NPSH

Please go through the below link for more information about NPSH

Formulas of pump NPSH and head loss calculation in suction and delivery line

Affinity laws for pumps

Change in Diameter

$\frac{ D_1}{D_2} = \frac{Q_1}{Q_2} = \frac{ \sqrt H_1}{\sqrt H_2}$

$\frac{ P_1}{P_2} = \frac{(D_1)^3}{(D_2)^3}$

D = Diameter of the impeller (inch or mm)

Q = Flow rate (gpm or m³/hr)

H = Head (ft or m)

P = Power ( hp or kW)

Change in Speed

$\frac{ N_1}{N_2} = \frac{Q_1}{Q_2} = \frac{ \sqrt H_1}{\sqrt H_2}$

$\frac{ P_1}{P_2} = \frac{(N_1)^3}{(N_2)^3}$

N = Pump speed (RPM)

Q = Flow rate (gpm or m³/hr)

H = Head (ft or m)

P = Power ( hp or kW)

Please go through the below link for more information about affinity laws

Affinity laws , Affinity laws for centrifugal pumps & Positive displacement pump with example

Pump Efficiency & Overall Efficiency of the Pump

Generally for any system efficiency means the ratio of output and input

Efficiency of the system = $\frac{Output }{Input }$
Pump Efficiency can be defined as a ratio of pump input and output power.

i.e Efficiency of the pump defined as the ratio of water horse power to break horse power.

$Pump \ Efficiency =\frac{Pump \ Output}{Pump \ Input} \ \times \ 100 \$

$Pump \ Efficiency = \frac{Water \ Horse \ Power}{Break \ Horse \ Power} \ \times 100 \$

$Pump \ Efficiency = \frac{Hydraulic \ Power}{Pump \ Shaft \ Power} \times 100$

Hydraulic power in Watt = $Q \ \times H \ \times\ \rho \ \times \ g$

Here

Q = Flow rate in m³/sec

H = Total developed head in meters

$\rho$ = Density in kg/m³

Hydraulic power in kW = $\frac{ Q \ \times H \ \times\ \rho \ }{ 102 \ }$

Here

Q = Flow rate in Lt./sec ( 1 m³/sec = 3.6 x Lt./sec)

H = Total developed head in meters

$\rho$ = Density in kg/dm³ (Conversion : 1 kg/m³ = 0.001 kg/dm³)

Pump shaft power means it is an input to pump often is the output of motor.

In case of gear drive or pulley drive, efficiency of these drives will also have to be taken into account

Output of motor = (kW input to motor) x motor efficiency (η_m)

Input to motor is measured directly in kW.

Then
Pump efficiency η_p = $\frac{ Q \ \times H \ \times\ \rho \ }{ 102 \time \ kW \ input \ to \ motor \time \ \eta_m }$

Overall Efficiency of the pumping system

Overall efficiency (η_overall) = Pump efficiency (η_p) x Motor efficiency (η_m)

Overall efficiency (η_overall) = $\frac{ Q \ \times H \ \times\ \rho \ }{ 102 \time \ kW \ input \ to \ motor \ }$

Related Articles:

Classification of pumps | Types of pumps and their working principles

NPSH Calculation |Head loss in suction and delivery line

Pump Vapour pressure calculation | Water Vapour Pressure Table at Different temperatures

Affinity Laws for Centrifugal and Positive displacement pumps

Pump Efficiency and Pump Power Calculation Formulas with Online Calculator

Pressure head, Velocity Head formulas with examples

Unit Conversion Factors and Tables for Engineering Design Calculations

3 thoughts on “Pump Related formulas | Power calculation, Total Head, NPSH, Affinity laws”

joel

(September 20, 2022 - 5:11 am)

very handy to speed up calculation

siva alluri

(October 1, 2022 - 3:31 pm)

welcome

Debasis Maitra

(December 8, 2022 - 11:07 am)

Can we calculate head of a pump with the the help of impeller diameter or any other simple method for pump discharge head calculation.

Pump Related formulas | Power calculation, Total Head, NPSH, Affinity laws

Basic required formulas for the operation of Pump

Formulas regarding product while pumping

Pump Power Calculation

Specific Speed of Pump (Nq)

Total Head

Pump Torque and temperature rise

Net Positive Suction Head

Affinity laws for pumps

Pump Efficiency & Overall Efficiency of the Pump

Post Author: siva alluri

Related Posts

3 thoughts on “Pump Related formulas | Power calculation, Total Head, NPSH, Affinity laws”

joel

siva alluri

Debasis Maitra

Leave a Reply Cancel reply